
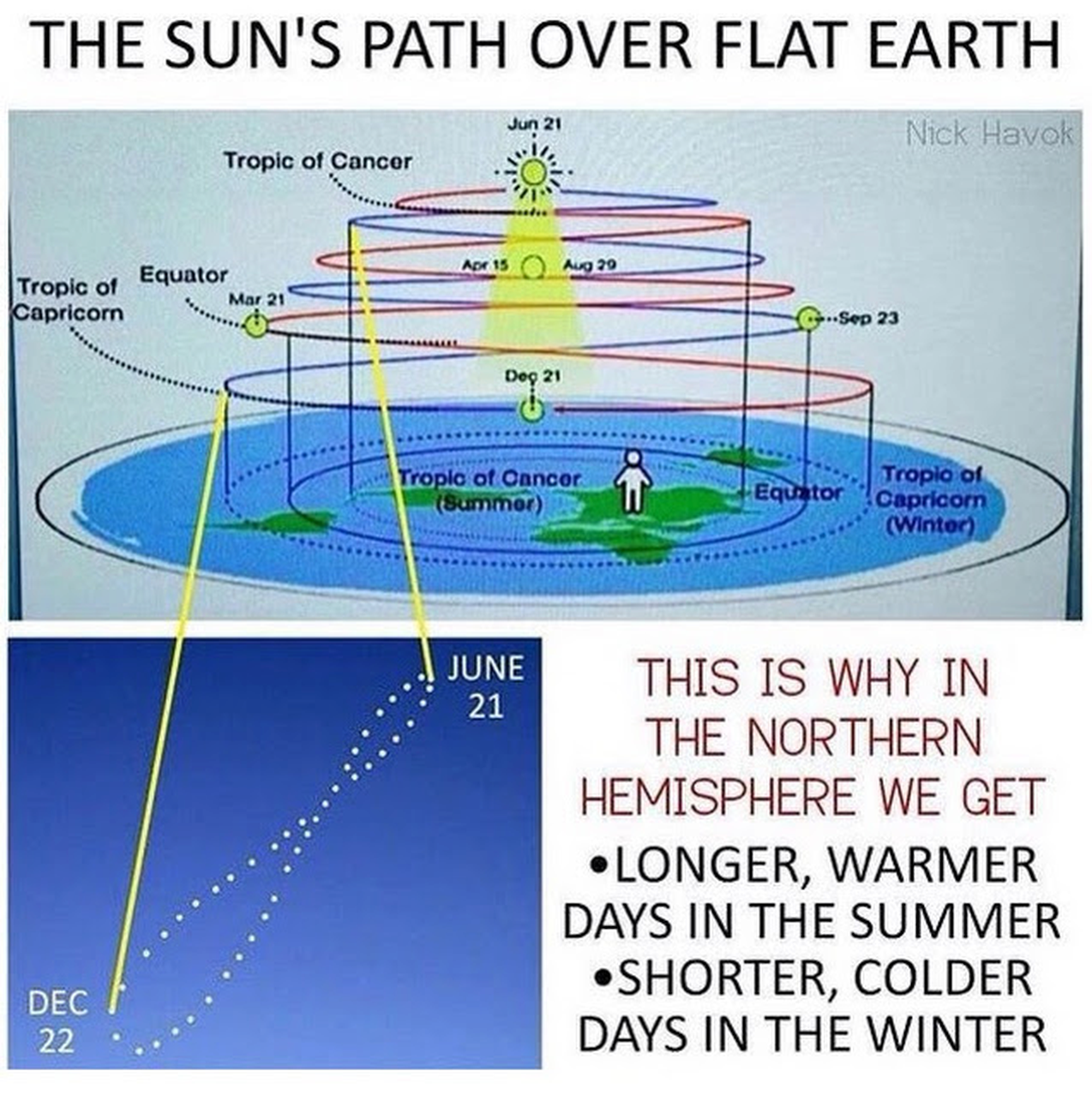
Remembering what happened with the Play-Doh, students can try to think of something similar that might be happening with the soap bubbles. No cubical bubbles, no pyramids, no pancake-shaped bubbles-just spheres! As soon as the bubble leaves the wand (or whatever gizmo they’re using to make the bubbles), the bubble immediately becomes round (see Figure 3). To see an example of this, students can blow some soap bubbles. So whenever a shape is squeezed together from all directions, it forms a sphere. They squeezed it from all directions (see Figure 2). Reflect on how students made the Play-Doh into a ball. You can also think of it as the most compact shape, with all parts of the Play-Doh as close to each other as they can get. Of all possible shapes-hot dogs, rings, cubes, irregular lumps-a sphere has the smallest amount of outside area. That’s the shape that has the least amount of surface area for any amount of Play-Doh. Let’s explore why that is.Īs students try to reduce the surface area, what you should observe is that-in an attempt to make the surface as small as possible-they squeeze their Play-Doh into a shape like a ball (or sphere), which is the correct answer. This is nonsense, of course, and there’s one particular scientific fact that they aren’t aware of: Regardless of the observations and how we may or may not be misled by them, the Earth MUST be round! The laws of physics require it. These “flat-Earthers” try to argue that a flat Earth can explain all of our observations and, at the same time, they claim that photos and video of the Earth taken from space showing a rotating, spherical Earth are all fakes. That shows a major failure in our educational system.
You might have noticed that there are some people who question whether the Earth is really round, or who actually believe (or claim to believe) that it’s flat. For the youngest students, we could ask, “Why is the Earth shaped like a ball?” A:Yes, I know, the question should really have been, “Why is the Earth approximately spherical?” The word round is a bit ambiguous, as it could refer to a two-dimensional circle or a three-dimensional sphere.


 0 kommentar(er)
0 kommentar(er)
